Data Analysis

Measurement of viscoelastic properties changes

During a DAET test, the low frequency palpation (LF) changes the propagation velocity and the amplitude of the ultrasound pulses (S).

Modulation of time of flight (TOFM) and relative amplitude modulation (RAM) of US pulses, during the various states of expansion/compression imposed by the LF wave, provides information on the compression modulation of viscoelastic parameters of the medium.

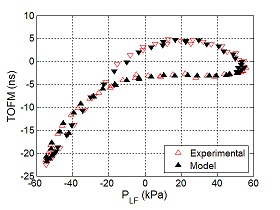
Acoustical Rheogram Modeling

RheaWave has developed a non-linear viscoelastic model to estimate relevant parameters from the experimental acoustic rheograms, using the non-linearity and the viscoelasticity of the medium.

For industrial applications, they will be used as a damage index or viscoelasticity/texture score.

What does the DAET technology measure?

In homogeneous elastic mediums, solid or liquid, the nonlinear parameters are characteristic of the composition of the medium and of its overall rigidity.

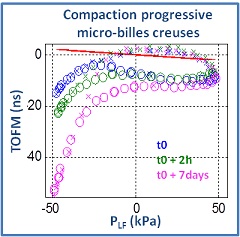
On the other hand, responses are very different if the medium has some inhomogeneities (micro-cracks, damage and contacts between grains) or different compressibility phases (air/fluid, air/solid).

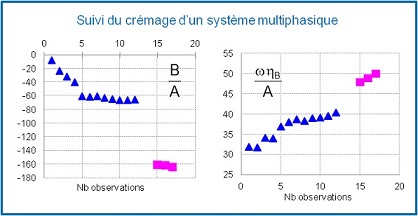
In the case of an evolving medium, time tracking of viscoelastic scores or index gives information about the progress of the phenomenon. This figure quantifies the creaming of a hollow microsphere solution, by recording the first two viscoelastic parameters over time.